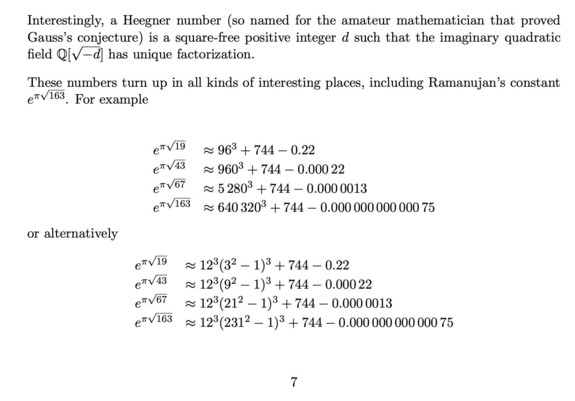
The fascinating Heegner numbers [1] are so named for the amateur mathematician who proved Gauss' conjecture that the numbers {-1, -2, -3, -7, -11, -19, -43, -67,-163} are the only values of -d for which imaginary quadratic fields Q[√-d] are uniquely factorable into factors of the form a + b√-d (for a, b ∈ ℤ) (i.e., the field "splits" [2]). Today it is known that there are only nine Heegner numbers: -1, -2, -3, -7, -11, -19, -43, -67, and -163 [3].

Interestingly, the number 163 turns up in all kinds of surprising places, including the irrational constant e^{π√163} ≈ 262537412640768743.9999999999992500... (≈ 2.6253741264×10^{17}), which is known as the Ramanujan Constant [3].

Some of my notes are here: https://davidmeyer.github.io/qc/galois_theory.pdf. As always, questions/comments/corrections/* greatly appreciated.

#math #galois #gauss #heegnernumber #ramanujan

References
--------------
[1] "Heegner Number", https://mathworld.wolfram.com/HeegnerNumber.html

[2] "Splitting Field", https://mathworld.wolfram.com/SplittingField.html

[3] "Heegner numbers: imaginary quadratic fields with unique factorization (or class number 1).", https://oeis.org/A003173

[4] "Ramanujan Constant", https://mathworld.wolfram.com/RamanujanConstant.html