⎯ΘωΘ⟶ · @yog
203 followers · 852 posts · Server mathstodon.xyzI have neglected this channel, sorry lol. If you follow me you will probably find this interesting: https://arxiv.org/abs/2307.00442
#qft #gaugetheory #motives #homotopy #categories
Atex · @Atexjam
957 followers · 6984 posts · Server mastodon.worldhxameer :qed: · @xameer
180 followers · 3838 posts · Server mathstodon.xyznot all equality was proven using reflexivity. My understanding of the matter is that is has to do with the placement of the forall (x : A) quantifier. It is permissible to move one of the x's to the top level (based path induction), but not both. (This is somewhat obscured by the reuse of variable names.) There is also a geometric intuition, which is that when both or one endpoints of the path are free (inner-quantification), then I can contract the path into nothingness. But I have a difficult time mapping this onto any sort of rigorous argument.
http://blog.ezyang.com/2013/06/homotopy-type-theory-chapter-one/
#homotopy folks any clue on it?
RanaldClouston · @RanaldClouston
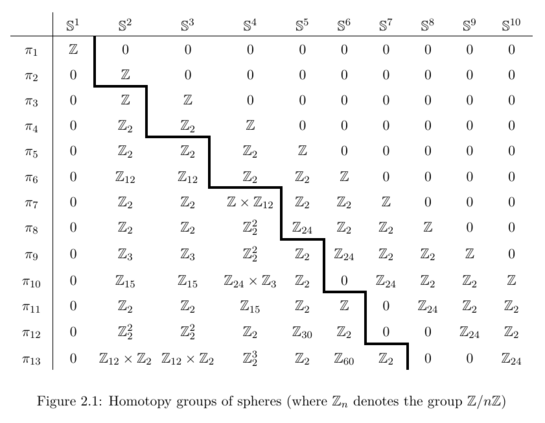
204 followers · 641 posts · Server fediscience.orgOn this week's #blog , a bit late as I work around the start of teaching this semester, I write about the fascinating PhD thesis, 'On the homotopy groups of spheres in homotopy type theory' https://updatedscholar.blogspot.com/2023/02/discussing-on-homotopy-groups-of.html #Hott #TypeTheory #Homotopy
#homotopy #typetheory #hott #blog
Charlotte Kirchhoff-Lukat · @charlottekl
260 followers · 447 posts · Server mathstodon.xyz#Reference request: I am attending a learning seminar on #Floer #Homotopy theory this semester. Coming from the symplectic side of things, I could use a nice accessible reference on the #algebra side, specifically on stable ∞ -#categories and the category of spectra in particular.
I already have Chapter 1 of *Higher Algebra* by Jacob Lurie.
Anybody have any other good suggestions? (Ideally ones that do not require the whole kitchen sink of model categories.)
Thanks in advance! 🙂 :k5:
#categories #algebra #homotopy #floer #reference
Colin the Mathmo · @ColinTheMathmo
2317 followers · 16258 posts · Server mathstodon.xyz@buchholtz It's worth putting #HashTags in your posts to help people find relevant conversations:
Give it time, but people will find each other.
And welcome!
#typetheory #homotopy #hashtags
⎯ΘωΘ⟶ · @yog
203 followers · 852 posts · Server mathstodon.xyz#arbitrage #pricing #theory #decision #praxis #holonomy #information #manifold #curvature #noncommutative #geometry #modal #homotopy #type
#type #homotopy #modal #geometry #noncommutative #curvature #manifold #information #holonomy #praxis #decision #theory #pricing #arbitrage
⎯ΘωΘ⟶ · @yog
203 followers · 852 posts · Server mathstodon.xyzimagine a #modal #homotopy #type #theory based on those modalities: #knowledge, #belief, and #perception
#perception #belief #knowledge #theory #type #homotopy #modal
mug · @mugnaini
80 followers · 70 posts · Server mathstodon.xyzHello folks! I'm an undergraduate student in mathematics interested in abstract homotopy theory, and category theory at large! Aside from that, in my spare time I love programming, reading books and listening some good music!
I'm also into vegetarianism, philosophy, open source, looking forward to learn more about socialism and a variety of other topics :)
#maths #categoryTheory #homotopy #programming #music #books #openSource #philosophy #vegetarian #socialism
#socialism #vegetarian #philosophy #opensource #books #music #programming #homotopy #categorytheory #maths #introduction