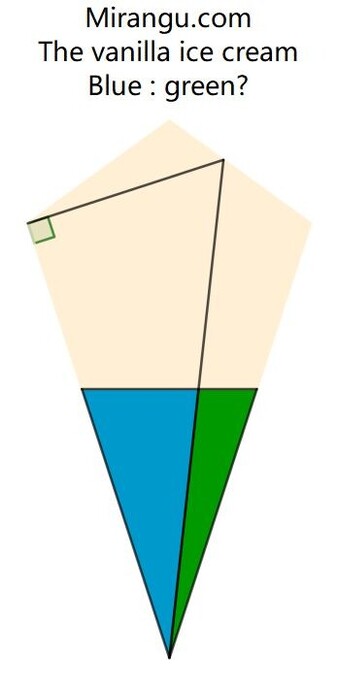
Benjamin Leis ✅ · @benleis
372 followers · 1026 posts · Server mathstodon.xyzThis recent one from @mirangu@twitter is fascinating because the regular pentagon means there will be phi in the workings.
Benjamin Leis ✅ · @benleis
361 followers · 957 posts · Server mathstodon.xyz@dhabecker I'm only not answering here because I did the problem originally when posted. It's a fun #MathPuzzle
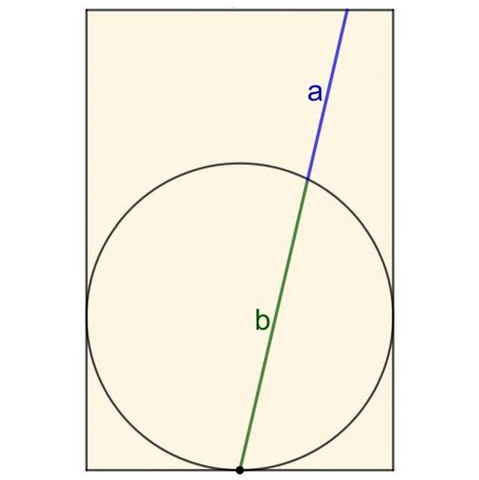
Benjamin Leis ✅ · @benleis
354 followers · 907 posts · Server mathstodon.xyzI like the problems where the setup is super simple but it seems like there isn't enough info to solve and yet ... there is.
via: @BehroozParhami@twitter
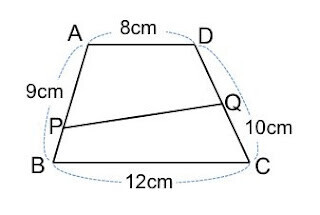
Benjamin Leis ✅ · @benleis
354 followers · 903 posts · Server mathstodon.xyzIf DQ is 5cm and the area of ADQP = area of BCQP what is the length of AP?
via @five_triangles@twitter #MathPuzzle #geometry
Benjamin Leis ✅ · @benleis
351 followers · 867 posts · Server mathstodon.xyzWhen is the following expression an integer?
\( \dfrac{6^{r+s} \cdot 12^{r-s}}{8^r \cdot 9^{r+2s}} \)
[@solvemymaths via MAT]
Benjamin Leis ✅ · @benleis
352 followers · 830 posts · Server mathstodon.xyz
Ilana Cyna · @ilanacyna
43 followers · 11 posts · Server mathstodon.xyz#MathPuzzle #ClassroomMath #prompt4
Not a specific puzzle being shared, but...
I like having challenges available for my students should they finish their task before others. Sometimes I give challenges related to the content of what we are doing, and other times they are more generic. I keep on hand booklets of logic puzzles from Puzzle Baron and Inkies from Crazy Dad (otherwise known as Ken Ken puzzles). For both, you can choose a variety of difficulty levels on their websites.
https://logic.puzzlebaron.com/
https://krazydad.com/inkies/
#prompt4 #classroommath #mathpuzzle
Benjamin Leis ✅ · @benleis
348 followers · 800 posts · Server mathstodon.xyzhttp://blog.mathoffthegrid.com/2019/05/langleys-adventitious-angles.html
Since @wtgowers was live solving Langley's Adventitious angles on youtube today here's my old post on it.
Hint: you need to add auxiliary lines. Try increasing the symmetry
Sam Shah · @samjshah
271 followers · 395 posts · Server mathstodon.xyz#MathPuzzle #prompt4 #ClassroomMath
I had so many problems that came to mind that I wanted to share... But I chose this problem because I wanted to share something that brought ME joy when I figured it out... but also something that I use in my class because of it.
It all started with "The Block Problem" which then led me to think about higher dimensional cubes which then led me to a chart... A chart that ended up holding lots of cool mysteries!
I always get to the point in my Adv. Precalculus classes where I get kids to answer the first question I pose in the blogpost below. So that's how I use this problem in class. But the second and third problems, I just really love. One year we solve them in class (the year I discovered them). But mainly I felt so proud of myself that I *could* solve them!
https://samjshah.com/2023/07/17/hypercubes-and-more-two-problems-you-may-enjoy-working-on/
#classroommath #prompt4 #mathpuzzle
Mark Kreie · @Kreiem
30 followers · 7 posts · Server mathstodon.xyz#MathPuzzle #prompt4 #ClassroomMath
I love this 3 ACT task and it is awesome for an 8th grade / pre-algebra / algebra 1 level class.
[Google Dan Meyer 3-ACT for some details about 3 ACT tasks if you need insight into how to execute this type of task. S/O to Andrew Stadel for the awesome task via 101qs.]
#classroommath #prompt4 #mathpuzzle
Benjamin Leis ✅ · @benleis
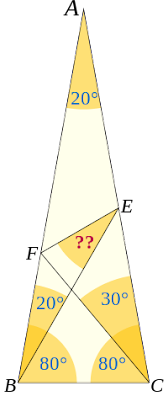
347 followers · 778 posts · Server mathstodon.xyzVariant on yesterdays problem that is fun in its own right and a lemma towards a synthetic proof.
Given 2 right isoscleses triangles. ABC and CDF hinged together at C, if you connect BD and form a another right isosceles triangle BDE, show ACFE is a parallelogram
Benjamin Leis ✅ · @benleis
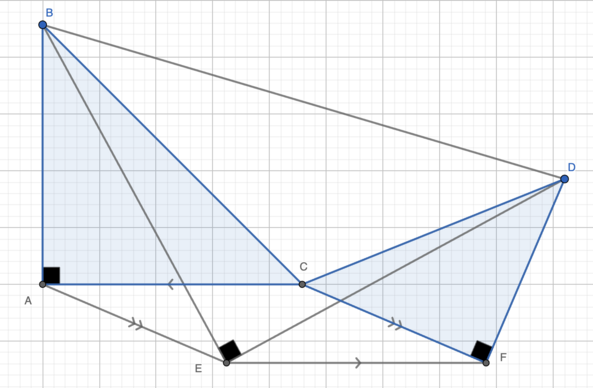
346 followers · 767 posts · Server mathstodon.xyzSince James Tanton isn't here and this problem is quite interesting:
*Two isosceles right triangles are hinged at corners as shown. Line segment connecting midpoints of their hypotenuses is used as the hypotenuse of yet another isosceles right triangle.
Prove A, B, C lie on a straight line.
Can anything be said about where B sits on segment AC?". #MathPuzzle #geometry
Julie Reulbach · @jreulbach
245 followers · 169 posts · Server mathstodon.xyzToday, my math peeps, we’re going to be sharing and maybe doing math! Is there a problem that you love having your kids work on? A favorite problem that evokes conversation and ah hah moments? Or is there a math problem or puzzle you’ve seen or done that made you go “oh, wow!”?
In your post:
1. Write down your math problem! If you use it in your class, share the grade/class you use it with, and why you like the problem for your kids. If it’s just a recreational math problem or puzzle, feel free to just drop it in the post. You can decide whether you want to give a hint or not! (NOTE: you can use the “CW” button when writing a post to hide the post’s content until someone clicks on it… so you can type the problem in one post, and then in a reply to that post, you can use “CW” to hide the hint until someone decides they want to see it.)
2. Tag your post with #MathPuzzle and #prompt4 and #ClassroomMath
Don’t forget to bookmark any problem/puzzle you might want to use in the future!
To practice using the site, we have just one challenge for you: practice using the fancy math ability of mathstodon to write an equation in a post. The fancy math equations only show up when you read posts on the desktop (not in apps, yet…), but it’s pretty awesome.
From the desktop page, click on the “f(x)” button and click “inline equation.” Some slashes and parentheses will appear. In between those, type:
\[ g(x)=\frac{\sqrt{3x-5}}{3}+\frac{x^{52}-x}{3\pi \sin^{2}(x)}-5x^{6}+a_{n} \]
See what happens! Believe it or not, you’ve typed pretty LaTex math.
#mathpuzzle #prompt4 #classroommath
Sam Shah · @samjshah
263 followers · 373 posts · Server mathstodon.xyzToday, my math peeps, we’re going to be sharing and maybe doing math! Is there a problem that you love having your kids work on? A favorite problem that evokes conversation and ah hah moments? Or is there a math problem or puzzle you’ve seen or done that made you go “oh, wow!”?
In your post:
1. Write down your math problem! If you use it in your class, share the grade/class you use it with, and why you like the problem for your kids. If it’s just a recreational math problem or puzzle, feel free to just drop it in the post. You can decide whether you want to give a hint or not! (NOTE: you can use the “CW” button when writing a post to hide the post’s content until someone clicks on it… so you can type the problem in one post, and then in a reply to that post, you can use “CW” to hide the hint until someone decides they want to see it.)
2. Tag your post with #MathPuzzle and #prompt4 and #ClassroomMath
Don’t forget to bookmark any problem/puzzle you might want to use in the future!
To practice using the site, we have just one challenge for you: practice using the fancy math ability of mathstodon to write an equation in a post. The fancy math equations only show up when you read posts on the desktop (not in apps, yet…), but it’s pretty awesome.
From the desktop page, click on the “f(x)” button and click “inline equation.” Some slashes and parentheses will appear. In between those, type:
g(x)=\frac{\sqrt{3x-5}}{3}+\frac{x^{52}-x}{3\pi \sin^{2}(x)}-5x^{6}+a_{n}
See what happens! Believe it or not, you’ve typed pretty LaTex math.
#mathpuzzle #prompt4 #classroommath
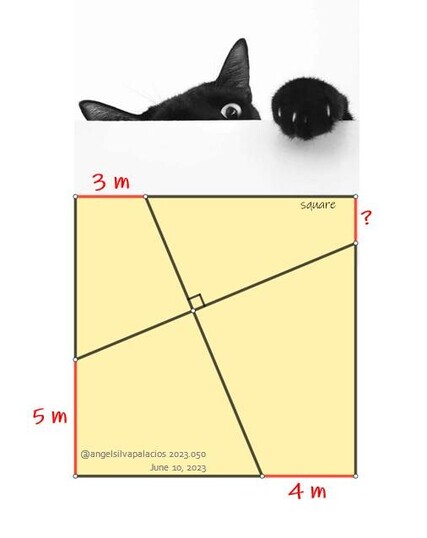
Benjamin Leis ✅ · @benleis
346 followers · 764 posts · Server mathstodon.xyzBenjamin Leis ✅ · @benleis
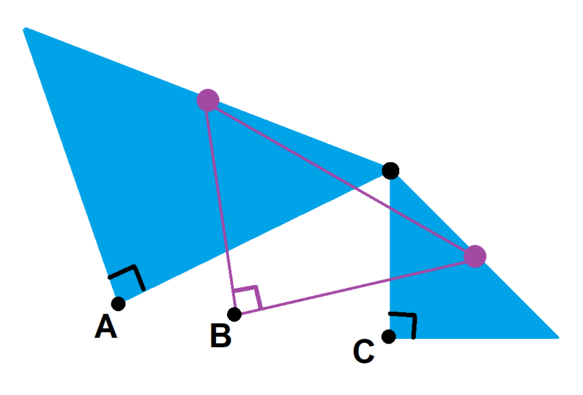
346 followers · 764 posts · Server mathstodon.xyzA classic area puzzle setup.
Benjamin Leis ✅ · @benleis
343 followers · 753 posts · Server mathstodon.xyz@aksharvarma only because you can't do boolean hashtag searches I've been using #MathPuzzle
Benjamin Leis ✅ · @benleis
340 followers · 737 posts · Server mathstodon.xyzThis one's quite a bit more complicated than the last problem I posted.
I thought it might make a good walk through sometime soon although I wouldn't do it live ala @DavidKButler as my own process is both more interrupted and sometimes takes a few days for complicated things.
via [@EylemGeryk]
Benjamin Leis · @benleis
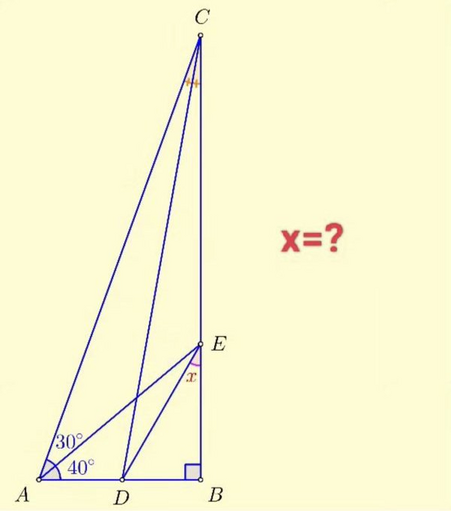
333 followers · 699 posts · Server mathstodon.xyzThat last problem (https://mathstodon.xyz/@benleis/110680805281317873) reminded me a bit in structure of this one which has a similar twist and might make a good warm up if you haven't tried it yet.
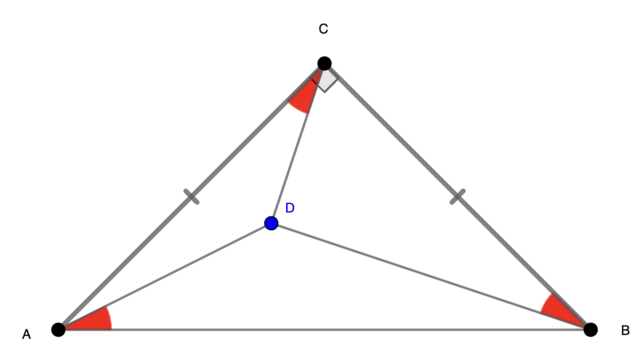
Given a right isosceles triangle ABC with three congruent angles ACD, CBD and BAD of measure θ that meet at point P find the tangent of θ .
I'm not quite sure its there but extra credit for finding a hidden 3-4-5
Benjamin Leis · @benleis
329 followers · 687 posts · Server mathstodon.xyzI was thinking about the excellent gogeometry.com site. This is the problem that I first tried n years ago. It looks simple but I spent a week at it.