Richard Penner · @Arpie4Math
48 followers · 119 posts · Server mathstodon.xyz@noswald An #AlgebraicCurve is named by the order of the implicit polynomial equations describing them.
\[ \frac{1}{x^2} + \frac{1}{y^2} = 1 \Rightarrow x^2 y^2 - x^2 - y^2 = 0 \]
So it is a #quartic curve of the form 𝑥²𝑦²−𝑏²𝑥²−𝑎²𝑦²=0 which is called a #cruciform curve, cross curve, or sometimes the policeman on point duty curve.
https://mathworld.wolfram.com/Cruciform.html
https://en.wikipedia.org/wiki/Quartic_plane_curve#Cruciform_curve
#cruciform #quartic #algebraiccurve
Rick Rae · @RickRae
53 followers · 90 posts · Server fosstodon.org@orci @Raspberry_Pi Ditto; it was invaluable at the time and I can't bear to part with an old friend.
But in these days of ever-present smartphones, I use Quartic's RealCalc in RPN mode instead. Feels like "home."
Never could get any of my friends to see the beauty in RPN, though...
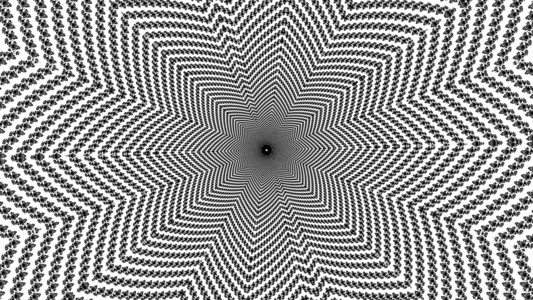
claude · @mathr
288 followers · 2739 posts · Server post.lurk.org#quartic (power 4) #BurningShip #fractals #art #monochrome #opart
a = 0.639842372544549201204928357495775021977648268509402840359034
b = 0.135996460127830318428355021688587977964732034634690759751358
s = 5.7332335426957978e-43
t = (0.6928120449739648,-0.713231167787216,0.7163656381606373,0.7059139674028518)
#quartic #burningship #fractals #art #monochrome #opart