Here is a close view of some of the #rhombusRosette bit.

Related to the rotating 2n-fold polygons to get rhombus rosettes-thing, here is a picture involving rotating partial rings of polygons to get…well…something.

It is from about 3 years ago. #rhombusRosette #polygons

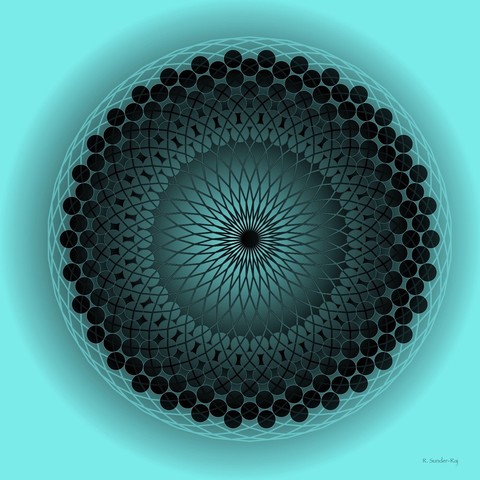
A 22-fold rotationally-symmetric thing with most of a 22-fold rhombus rosette in it.

#mathart #mathsart #rhombuses #rhombi #rhombusRosette #symmetry

An 11-fold rotationally-symmetric thing with a 22-fold rhombus rosette in it.

#mathart #mathsart #rhombuses #rhombi #rhombusRosette #symmetry

This one may be a bit more interesting. It is an attempt to decompose simple n-fold rhombus rosettes into smaller rosettes in a way that is related to the factors of n.

#rhombusRosette

Woven #rhombusRosette stuff

#mathart #woven

Clips from the rhombusworm video.

The first shows equilateral (but not necessarily equiangular) polygons rotating to form n-fold rhombus rosettes. In particular, if n is even, then they can be generated by rotating regular n-gons around a point, but if n is odd, it can work with somewhat squished (n+1)-gons formed by merging two equilateral n-gons in a particular way.

The second shows rhombusworms rotating to form the same rosettes.

#rhombusworms #polygons #rhombusRosette #regularPolygons