Paysages Mathématiques · @paysmaths
141 followers · 646 posts · Server mathstodon.xyzFamous mathematical theorems illustrated with Midjourney.
Source : Twitter / Tivadar Danka
https://twitter.com/TivadarDanka/status/1649721970886594561
#mathematics #math #maths #theorems #Midjourney
#midjourney #theorems #maths #math #mathematics
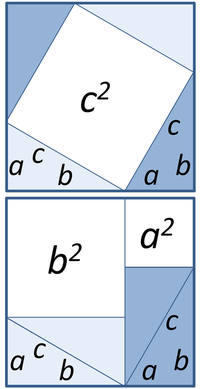
Knowledge Zone · @kzoneind
97 followers · 303 posts · Server mstdn.social#KnowledgeByte: #Theorems, #Lemmas, #Conjectures and more - Some common definition and brief concepts. #Math101
#math101 #conjectures #lemmas #theorems #knowledgebyte
OCFelisola · @OCFelisola
20 followers · 158 posts · Server mathstodon.xyzAfter my previous reply to @erou I remember a couple of *my early theorems*, at the time (10-12 years old) I knew the results were right, but the actual proof come much later when I was in the mid of my B.Sc.
1. \(\frac{1}{2} + \frac{1}{4} + \frac{1}{8} + \cdots = \sum_{n=1}^\infty 2^{-n} = 1\).
2. \(\frac{1}{2} - \frac{1}{4} + \frac{1}{8} - \frac{1}{16} + \cdots = \sum_{n=1}^\infty (-1)^{n-1} 2^{-n} = \frac{1}{3}\).
#science #young #theorems #mathematics
OCFelisola · @OCFelisola
20 followers · 158 posts · Server mathstodon.xyz@erou Wow! I solve that problem (intuitively) when I was like 10 years old (I had a notebook with my theorems... there were about three! five tops, but I was really proud).
Back then I noted that any \(n\)-polygon can be split into \(n-2\) triangles (much later, I found that it is the base of triangulation XD), from there the result is trivial.
#science #young #theorems #mathematics
Ian Kenway · @iankenway
266 followers · 4667 posts · Server qoto.org#mathematics #equations #theorems #fractals #chaos_theory #google #youtube #sumpter #uppsala #sweden #global
Equations built giants like Google. Who’ll find the next billion-dollar bit of maths?
#mathematics #equations #theorems #fractals #chaos_theory #google #youtube #sumpter #uppsala #sweden #global